Boas–Buck polynomials

In mathematics, Boas–Buck polynomials are sequences of polynomials Φ(r)
n(x) given by generating functions of the form

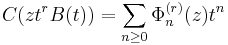$\displaystyle C(zt^r B(t))=\sum_{n\ge0}\Phi_n^{(r)}(z)t^n$ .

The case r=1, sometimes called generalized Appell polynomials, was studied by Boas and Buck (1958).

References

Boas, Ralph P.; Buck, R. Creighton (1958), Polynomial expansions of analytic functions, Ergebnisse der Mathematik und ihrer Grenzgebiete. Neue Folge., 19, Berlin, New York: Springer-Verlag, MR 0094466, http://books.google.com/books?id=eihMuwkh4DsC